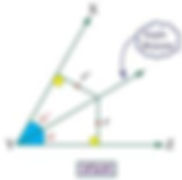
An angle bisector is a ray that divides an angle into two equal halves. More formally, if ray BD is the bisector of ∠ABC, then ∠ABD = ∠DBC, and ∠ABC is divided into two congruent angles. This article provides a detailed proof of this fundamental definition of an angle bisector.
The Significance of Angle Bisectors
Understanding angle bisectors is key in geometry for numerous applications such as finding hidden vertices in isosceles and equilateral triangles, locating circumcenters of triangles, and providing equality conditions to solve problems. By proving why an angle bisector divides the angle into exactly two congruent halves, we build intuition for using this powerful concept.
Furthermore, employing a unit circle chart can serve as a valuable tool in visualizing and reinforcing the principles associated with angle bisectors.
Key Terminology in This Article
Angle, Ray, Congruent Angles, Triangle, Isosceles Triangle, Equilateral Triangle, Circumcenter
Visualizing the Angle Bisector
Consider ∠ABC defined by rays AB and AC with vertex at point B. The angle bisector BD is a ray originating at vertex B and intersecting AC at point D such that ∠ABD = ∠DBC. Visualizing this relationship is pivotal before proving it mathematically.
Labeling Key Points on the Diagram
Mark points A, B and C defining ∠ABC. Draw BD to bisect the angle such that it divides ∠ABC into two smaller angles - label them ∠ABD and ∠DBC. Highlight point D as the intersection of BD and ray AC.
Relating Circumcenter Concept
Interestingly, D also denotes the circumcenter of ΔABD, locating equidistance from its vertices. This connection will be leveraged later in the equivalence proof.
Constructing a Formal Proof
We will now construct a formal geometric proof by contradiction to show that ray BD drawn as the angle bisector ensures ∠ABD and ∠DBC are congruent angles.
Assume the Contrapositive
The proof starts by assuming the contrapositive - that ∠ABD and ∠DBC are NOT congruent for the arbitrarily drawn BD.
Apply Triangle Equidistance Principle
Since D is the circumcenter of △ABD, by definition, AD = BD = DC.
Use SAS Triangle Congruence
By the SAS congruence condition, if two triangles have two corresponding sides (AD = DC from above) and the included angle equal (∠ADC is common), then the triangles are congruent.
Arrive at A Contradiction
Since △ABD ∼ △BCD by SAS but our assumption states ∠ABD ≠ ∠BDC, this is a contradiction.
Conclude the Proof
Thus the assumption “ray BD not bisecting ∠ABC” must be incorrect. Therefore, BD drawn as angle bisector necessarily makes ∠ABD ≅ ∠DBC, proving the fundamental definition.
Analyzing Special Cases
The above proof can further be extended to validate special cases like angle bisectors in isosceles and equilateral triangles using additional congruence conditions.
Isosceles Triangles
In an isosceles triangle with AB = AC, by drawing AD⊥BC, D becomes both the angle bisector and perpendicular bisector, splitting ∠B and BC into two congruent halves.
Equilateral Triangles
Every angle bisector drawn from a vertex to the opposite side also becomes an altitude, median, and perpendicular bisector in an equilateral triangle since all sides and angles are equal.
Applications of Angle Bisectors
Having rigorously proven the foundational definition, let us explore some common applications of angle bisectors.
Locating Circumcenter of Triangles
As shown earlier, the angle bisector vertices in any triangle denote their circumcenters. So angle bisectors locate triangles' circumcenters.
Solving Geometric Constructions
Angle bisector lengths or ratios help calculate unknown values in multi-step constructions and coordinate geometry problems to arrive at solutions.
Finding Locus Points in Complex Figures
In complex geometric shapes with multiple triangles, judiciously constructing angle bisectors helps determine useful points like incenters and centroids through their intersections.
Conclusion
Through this comprehensive proof analysis, the fundamental concept defining an angle bisector to split any angle exactly into two congruent halves has been established. By leveraging circumcenter properties and contradiction logic, the equality of the sub-angles formed has been proven beyond doubt.
Internalizing this foundational proof will make applying angle bisector properties effortless during problem solving across geometry and trigonometry. Grasping how angle bisectors provide shortcuts to locating special points will also be much more intuitive.
With the insight gained from this article, you are now fully equipped to leverage angle bisectors confidently in your math learning journey!
Can any angle be bisected?
Yes, any angle defined with distinct rays and a common vertex can be bisected by drawing an angle bisector ray that splits the angle exactly into two congruent sub-angles. There are no constraints on minimum/maximum size.
Are an angle bisector and perpendicular bisector the same?
No, an angle bisector specifically splits the angle into two equal angles. A perpendicular bisector divides any one side of the triangle into two equal halves by drawing a perpendicular from the opposite vertex. The two are equal only in isosceles triangles.
What is the shortest path to bisect an angle?
The shortest line segment bisecting an angle is the one that originates from the angle vertex and intersects the angle exactly at its midpoint. This path to bisect ensures minimum distance while splitting congruently.
